BrainVoyager v23.0

EEG/MEG Continous Wavelet Filtering

In order to analyze the oscillatory activity from EEG or MEG data sets across separate epochs, a continous wavelet transform (CWT) method can be conveniently used in the context of a cortically distributed source EEG or MEG analysis. During source time-course reconstruction, the estimated inverse solution (spatial filger) can be applied to CWT filtered single-trial data (STD) data sets from a compatible configuration. In this case, after averaging across all trials, the reconstructed source time-courses will represent either the perturbation in the source spectral power at a given frequency (default) or the corresponding phase-locking (or inter-trial coherence) evolution induced by a triggering event (see chapter on Source time course reconstruction).

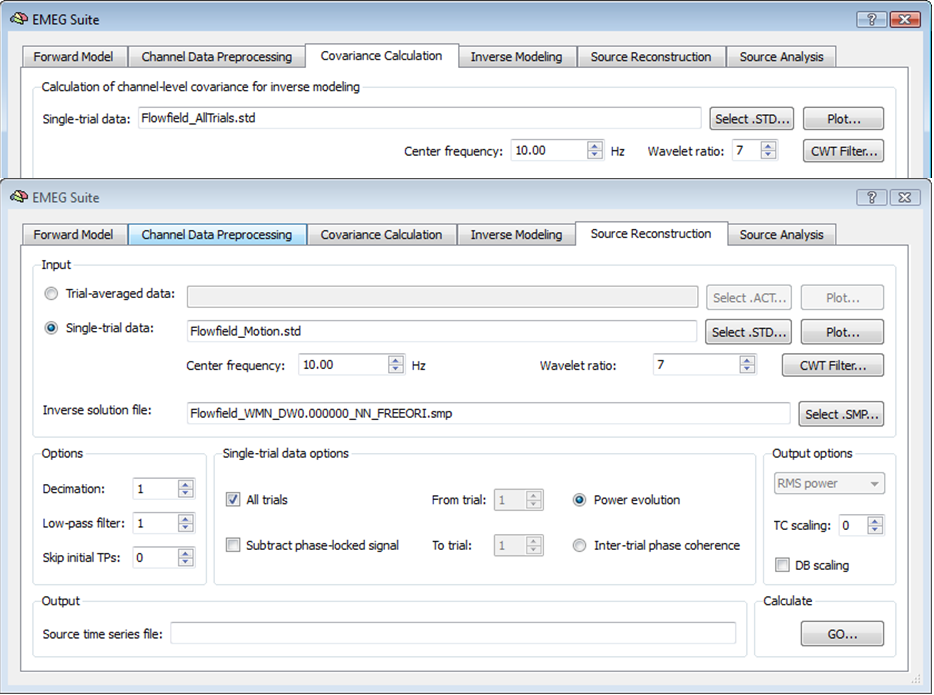
The CWT filtering of the EEG MEG single-trial signals can be performed either at the Covariance stage (3rd tab of the EMEG suite) or right before proceeding with the source time-course reconstrution (4th tab of the EMEG suite). During CWT filtering, each single epoch j of each single channel i of the measurement (mij) from the single-trial data set (STD) is temporally convolved with the complex Mortlet's wavelet (y) centered at the specified center frequency fc and with the specified wavelet ratio w:

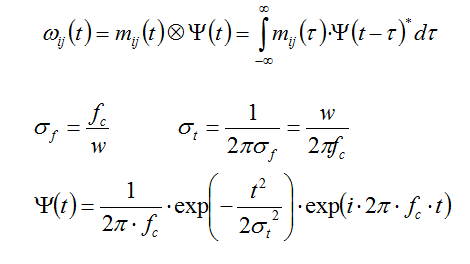
The complex Mortlet's wavelet corresponds to a complex exponential wavelet (centered at frequency fc) modulated by a Gaussian function with standard deviation inversely proportional to the bandwith of the complex exponential wavelet. The wavelet ratio allows controlling the actual frequency bandwith of the trasfer function in relation to the center frequency and consequently the temporal duration of the wavelet.

For example, selecting 40Hz signals (fc=40) with w=7 will give approximately give sf=5.71 Hz and st~28ms. These values allow giving an estimate of the actual time-frequency resolution of this transfom in terms of the frequency and temporal standard deviations of the wavelet. For a given center frequency, the bandwidth of the filtering can be reduced (and the frequency filtering made more selective) by increasing the wavelet ratio, however this proportionality automatically increase the standard duration of the wavelet. This poses some fundamental limitations to the possibility of filtering a certain effect in the time-frequency domain via a CWT of any STD. In fact, the higher the frequency selectivity of the filtering, the longer the trial period needs to be for allowing an adequate coverage of the temporal evolution of the effect. Thefore, after setting the center frequency and the wavelet ratio, running the CWT filter will always start a check that the transform can be operated without problem and the user is required to confirm the settings in terms of an estimate of frequency bandwith and temporal duration given the sampling rate and the number of time points in the current STD.